LINE GRAPH

 MATHS & REASONING

The following line graph gives the ratio of the amounts of imports by a company to the amount of exports from that company over the period from 1995 to 2001.

Ratio of Value of Imports to Exports by a Company Over the Years.

1.	If the imports in 1998 was Rs. 250 crores and the total exports in the years 1998 and 1999 together was Rs. 500 crores, then the imports in 1999 was ?
	A. Rs. 250 crores B. Rs. 300 crores C. Rs. 357 crores D. Rs. 420 crores

---

2.	The imports were minimum proportionate to the exports of the company in the year ?
	A. 1995 B. 1996 C. 1997 D. 2000 Answer: Option C Explanation: The imports are minimum proportionate to the exports implies that the ratio of the value of imports to exports has the minimum value. Now, this ratio has a minimum value 0.35 in 1997, i.e., the imports are minimum proportionate to the exports in 1997.

---

3.	What was the percentage increase in imports from 1997 to 1998 ?
	A. 72 B. 56 C. 28 D. Data inadequate Answer: Option D Explanation: The graph gives only the ratio of imports to exports for different years. To find the percentage increase in imports from 1997 to 1998, we require more details such as the value of imports or exports during these years. Hence, the data is inadequate to answer this question.

---

4.	If the imports of the company in 1996 was Rs. 272 crores, the exports from the company in 1996 was ?
	A. Rs. 370 crores B. Rs. 320 crores C. Rs. 280 crores D. Rs. 275 crores Answer: Option B Explanation: Ratio of imports to exports in the year 1996 = 0.85. Let the exports in 1996 = Rs. x crores. Then, 272 = 0.85          x = 272 = 320. x 0.85  Exports in 1996 = Rs. 320 crores.

---

5.

In how many of the given years were the exports more than the imports ?

A. 1 B. 2 C. 3 D. 4 Answer: Option D Explanation: The exports are more than the imports imply that the ratio of value of imports to exports is less than 1. Now, this ratio is less than 1 in years 1995, 1996, 1997 and 2000. Thus, there are four such years. Study the following line graph and answer the questions based on it. Number of Vehicles Manufactured by Two companies ove the Years (Number in Thousands) 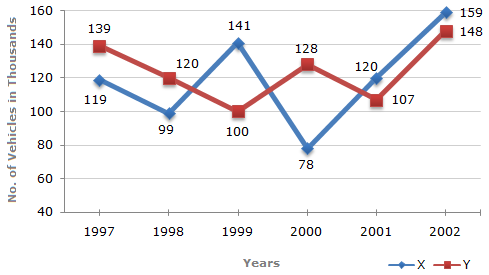1.  What is the difference between the number of vehicles manufactured by Company Y in 2000 and 2001 ? A. 50000 B. 42000 C. 33000 D. 21000 Answer: Option D Explanation: Required difference = (128000 - 107000) = 21000. 2.  What is the difference between the total productions of the two Companies in the given years ? A. 19000 B. 22000 C. 26000 D. 28000 Answer: Option C Explanation: From the line-graph it is clear that the productions of Company X in the years 1997, 1998, 1999, 2000, 2001 and 2002 are 119000, 99000, 141000, 78000, 120000 and 159000 and those of Company Y are 139000, 120000,100000, 128000, 107000 and 148000 respectively. Total production of Company X from 1997 to 2002     = 119000 + 99000 + 141000 + 78000 + 120000 + 159000     = 716000. and total production of Company Y from 1997 to 2002     = 139000 + 120000 + 100000 + 128000 + 107000 + 148000     = 742000. Difference = (742000 - 716000) = 26000. 3.  What is the average numbers of vehicles manufactured by Company X over the given period ? (rounded off to nearest integer) A. 119333 B. 113666 C. 112778 D. 111223 Answer: Option A Explanation: Average number of vehicles manufactured by Company X     = 1 x (119000 + 99000 + 141000 + 78000 + 120000 + 159000) 6     = 119333. 4.  In which of the following years, the difference between the productions of Companies X and Y was the maximum among the given years ? A. 1997 B. 1998 C. 1999 D. 2000 Answer: Option D Explanation: The difference between the productions of Companies X and Y in various years are: For 1997 (139000 - 119000) = 20000. For 1998 (120000 - 99000) = 21000. For 1999 (141000 - 100000) = 41000. For 2000 (128000 - 78000) = 50000. For 2001 (120000 - 107000) = 13000. For 2002 (159000 - 148000) = 11000. Clearly, maximum difference was in 2000. 5.  The production of Company Y in 2000 was approximately what percent of the production of Company X in the same year ? A. 173 B. 164 C. 132 D. 97 Answer: Option B Explanation: Required percentage = 128000 x 100 %  164%. 78000 The following line graph gives the percent profit earned by two Companies X and Y during the period 1996 - 2001. 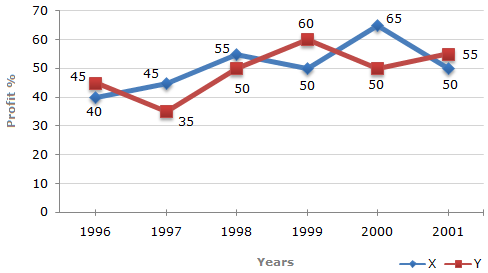Percentage profit earned by Two Companies X and Y over the Given Years %Profit = Income - Expenditure x 100 Expenditure 1.  The incomes of two Companies X and Y in 2000 were in the ratio of 3:4 respectively. What was the respective ratio of their expenditures in 2000 ? A. 7:22 B. 14:19 C. 15:22 D. 27:35 2.  If the expenditure of Company Y in 1997 was Rs. 220 crores, what was its income in 1997 ? A. Rs. 312 crores B. Rs. 297 crores C. Rs. 283 crores D. Rs. 275 crores 3.  If the expenditures of Company X and Y in 1996 were equal and the total income of the two Companies in 1996 was Rs. 342 crores, what was the total profit of the two Companies together in 1996 ? (Profit = Income - Expenditure) A. Rs. 240 crores B. Rs. 171 crores C. Rs. 120 crores D. Rs. 102 crores 4.  The expenditure of Company X in the year 1998 was Rs. 200 crores and the income of company X in 1998 was the same as its expenditure in 2001. The income of Company X in 2001 was ? A. Rs. 465 crores B. Rs. 385 crores C. Rs. 335 crores D. Rs. 295 crores 5.  If the incomes of two Comapanies were equal in 1999, then what was the ratio of expenditure of Company X to that of Company Y in 1999 ? A. 6:5 B. 5:6 C. 11:6 D. 16:15 The following line graph gives the percentage of the number of candidates who qualified an examination out of the total number of candidates who appeared for the examination over a period of seven years from 1994 to 2000. Percentage of Candidates Qualified to Appeared in an Examination Over the Years 1.  The difference between the percentage of candidates qualified to appeared was maximum in which of the following pairs of years? A. 1994 and 1995 B. 1997 and 1998 C. 1998 and 1999 D. 1999 and 2000 Answer: Option B Explanation: The differences between the percentages of candidates qualified to appeared for the give pairs of years are: For 1994 and 1995 = 50 - 30 = 20. For 1998 and 1999 = 80 - 80 = 0. For 1994 and 1997 = 50 - 30 = 20. For 1997 and 1998 = 80 - 50 = 30. For 1999 and 2000 = 80 - 60 = 20. Thus, the maximum difference is between the years 1997 and 1998. 2.  In which pair of years was the number of candidates qualified, the same? A. 1995 and 1997 B. 1995 and 2000 C. 1998 and 1999 D. Data inadequate Answer: Option D Explanation: The graph gives the data for the percentage of candidates qualified to appeared and unless the absolute values of number of candidates qualified or candidates appeared is know we cannot compare the absolute values for any two years. Hence, the data is inadequate to solve this question. 3.  If the number of candidates qualified in 1998 was 21200, what was the number of candidates appeared in 1998? A. 32000 B. 28500 C. 26500 D. 25000 Answer: Option C Explanation: The number of candidates appeared in 1998 be x. Then, 80% of x = 21200      x = 21200 x 100 = 26500 (required number). 80 4.  If the total number of candidates appeared in 1996 and 1997 together was 47400, then the total number of candidates qualified in these two years together was? A. 34700 B. 32100 C. 31500 D. Data inadequate Answer: Option D Explanation: The total number of candidates qualified in 1996 and 1997 together, cannot be determined until we know at least, the number of candidates appeared in any one of the two years 1996 or 1997 or the percentage of candidates qualified to appeared in 1996 and 1997 together. Hence, the data is inadequate. 5.  The total number of candidates qualified in 1999 and 2000 together was 33500 and the number of candidates appeared in 1999 was 26500. What was the number of candidates in 2000? A. 24500 B. 22000 C. 20500 D. 19000 Answer: Option C Explanation: The number of candidates qualified in 1999 = (80% of 26500) = 21200.  Number of candidates qualified in 2000 = (33500 - 21200) = 12300. Let the number of candidates appeared in 2000 be x. Then, 60% of x = 12300      x = 12300 x 100 = 20500. 60 The following line graph gives the annual percent profit earned by a Company during the period 1995 - 2000. Percent Profit Earned by a Company Over the Years. 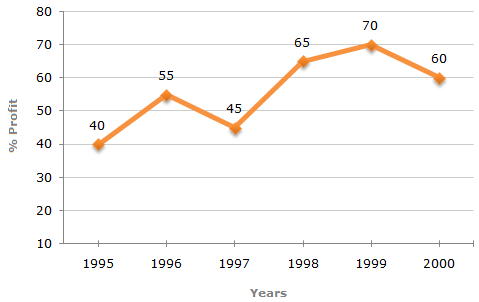%Profit = Income - Expenditure x 100 Expenditure 1.  If the expenditures in 1996 and 1999 are equal, then the approximate ratio of the income in 1996 and 1999 respectively is? A. 1:1 B. 2:3 C. 13:14 D. 9:10 Answer: Option D Explanation: Let the expenditure in 1996 = x. Also, let the incomes in 1996 and 1999 be I1 and I2 respectively. Then, for the year 1996, we have: 55 = I1 - x x 100      55 = I1 - 1      I1 = 155x ... (i) x 100 x 100 70 = I2 - x x 100      70 = I2 - 1      I2 = 170x ... (ii) x 100 x 100 From (i) and (ii), we get:     I1 = 155x 100 = 155 0.91  9 : 10. I2 170x 100 170 1 2.  If the income in 1998 was Rs. 264 crores, what was the expenditure in 1998? A. Rs. 104 crores B. Rs. 145 crores C. Rs. 160 crores D. Rs. 185 crores Answer: Option C Explanation: Let the expenditure is 1998 be Rs. x crores. Then, 65 = 264 - x x 100 x    65 = 264 - 1 100 x    x = 264 x 100 = 160. 165  Expenditure in 1998 = Rs. 160 crores. 3.  In which year is the expenditure minimum? A. 2000 B. 1997 C. 1996 D. Cannot be determined Answer: Option D Explanation: The line-graph gives the comparison of percent profit for different years bu the comparison of the expenditures is not possible without more data. Therefore, the year with minimum expenditure cannot be determined. 4.  If the profit in 1999 was Rs. 4 crores, what was the profit in 2000? A. Rs. 4.2 crores B. Rs. 6.6 crores C. Rs. 6.8 crores D. Cannot be determined Answer: Option D Explanation: From the line-graph we obtain information about the percentage profit only. To find the profit in 2000 we must have the data for the income or expenditure in 2000. Therefore, the profit for 2000 cannot be determined. 5.  What is the average profit earned for the given years? A. 50(2/3) B. 55(5/6) C. 60(1/6) D. 335 Answer: Option B Explanation: Average percent profit earned for the given years = 1 x [40 + 55 + 45 + 65 + 70 + 60] = 335 = 55 5 . 6 6 6 Answer the questions based on the given line graph. Ratio of Exports to Imports (in terms of money in Rs. crores) of Two Companies Over the Years 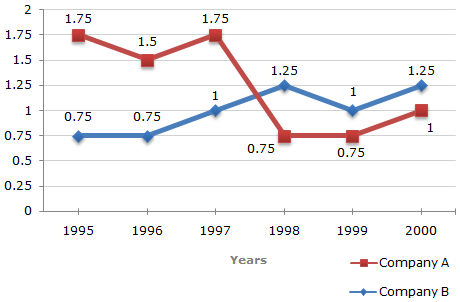1.  In how many of the given years were the exports more than the imports for Company A? A. 2 B. 3 C. 4 D. 5 Answer: Option B Explanation: The exports are more than imports in those years for which the exports to imports ratio are more than 1. For Company A, such years are 1995, 1996 and 1997. Thus, during these 3 years, the exports are more than the imports for Company A. 2.  If the imports of Company A in 1997 were increased by 40 percent, what would be the ratio of exports to the increased imports? A. 1.20 B. 1.25 C. 1.30 D. cannot be determined Answer: Option B Explanation: In 1997 for Company A we have: E = 1.75     i.e.,     E = 1.75I I where E amount of exports, I = amount of imports of Company A in 1997. Now, the required imports I1 = I + 40% of I = 1.4I.  Required ratio = E = 1.75I = 1.25. I1 1.4I 3.  If the exports of Company A in 1998 were Rs. 237 crores, what was the amount of imports in that year? A. Rs. 189.6 crores B. Rs. 243 crores C. Rs. 281 crores D. Rs. 316 crores Answer: Option D Explanation: Let the amount of imports of Company A in 1998 be Rs. x crores. Then, 237 = 0.75          x = 237 = 316. x 0.75  Amount of imports of Company A in 1998 = Rs. 316 crores. 4.  In 1995, the export of Company A was double that of Company B. If the imports of Company A during the year was Rs. 180 crores, what was the approximate amount of imports pf Company B during that year? A. Rs. 190 crores B. Rs. 210 crores C. Rs. 225 crores D. Cannot be determined Answer: Option B Explanation: In 1995 for Company A we have: EA = 1.75 ... (i) IA [where EA = amount of exports, IA = amount of imports of Company a in 1995] In 1995 for Company B we have: EB = 0.75 ... (ii) IB [where EB = amount of exports, IB = amount of imports of Company B in 1995] Also, we have EA = 2EB ... (iii) Substituting IA = Rs. 180 crores (given) in (i), we get:         EA = Rs. (180 x 1.75) crores = Rs. 315 crores. Using EA = Rs. 315 crores in (iii), we get:         EB = EA = Rs. 315 crores. 2 2 Substituting EB = Rs. 315 crores in (ii), we get: 2 IB = EB = Rs. 315 crores = Rs. 210 crores. 0.75 2 x 0.75 i.e., amount of imports of Company B in 1995 = Rs. 210 crores. 5.  In which year(s) was the difference between impors and exports of Company B the maximum? A. 2000 B. 1996 C. 1998 and 2000 D. Cannot be determined Answer: Option D Explanation: We shall try to find the difference between the imports and exports of Company B for various years one by one: For 1995: We have E = 0.75 I where E = amount of exports, I = amount of imports in 1995.  E = 0.75I  I - E = 0.75 x I = 0.25I. Thus, the difference between the imports and exports of Company B in 1995 is dependent on the amount of imports of Company B in 1995. Similarly, the difference for other years can be determined only if the amount of imports for these years is known. Since the imports or exports for various years are not know, the differences between and exports for various years cannot be determined. Two different finance companies declare fixed annual rate of interest on the amounts invested with them by investors. The rate of interest offered by these companies may differ from year to year depending on the variation in the economy of the country and the banks rate of interest. The annual rate of interest offered by the two Companies P and Q over the years are shown by the line graph provided below. Annual Rate of Interest Offered by Two Finance Companies Over the Years. 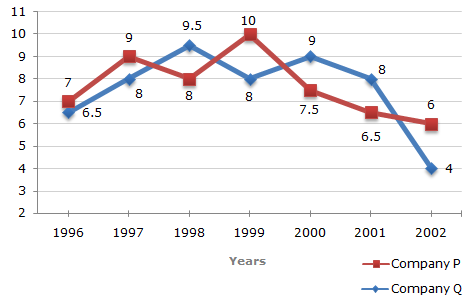1.  A sum of Rs. 4.75 lakhs was invested in Company Q in 1999 for one year. How much more interest would have been earned if the sum was invested in Company P? A. Rs. 19,000 B. Rs. 14,250 C. Rs. 11,750 D. Rs. 9500 Answer: Option D Explanation: Difference = Rs. [(10% of 4.75) - (8% of 4.75)] lakhs = Rs. (2% of 4.75) lakhs = Rs. 0.095 lakhs = Rs. 9500. 2.  If two different amounts in the ratio 8:9 are invested in Companies P and Q respectively in 2002, then the amounts received after one year as interests from Companies P and Q are respectively in the ratio? A. 2:3 B. 3:4 C. 6:7 D. 4:3 Answer: Option D Explanation: Let the amounts invested in 2002 in Companies P and Q be Rs. 8x and Rs. 9x respectively. Then, interest received after one year from Company P = Rs. (6% of 8x) = Rs. 48 x. 100 and interest received after one year from Company Q = Rs. (4% of 9x) = Rs. 36 x. 100  Required ratio = 48 x 100 = 4 . 36 x 100 3 3.  In 2000, a part of Rs. 30 lakhs was invested in Company P and the rest was invested in Company Q for one year. The total interest received was Rs. 2.43 lakhs. What was the amount invested in Company P? A. Rs. 9 lakhs B. Rs. 11 lakhs C. Rs. 12 lakhs D. Rs. 18 lakhs Answer: Option D Explanation: Let Rs. x lakhs be invested in Company P in 2000, the amount invested in Company Q in 2000 = Rs. (30 - x) lakhs. Total interest received from the two Companies after 1 year     = Rs. [(7.5% of x) + {9% of (30 - x)}] lakhs     = Rs. 2.7 - 1.5x lakhs. 100 2.7 - 1.5x = 2.43          x = 18. 100 4.  An investor invested a sum of Rs. 12 lakhs in Company P in 1998. The total amount received after one year was re-invested in the same Company for one more year. The total appreciation received by the investor on his investment was? A. Rs. 2,96,200 B. Rs. 2,42,200 C. Rs. 2,25,600 D. Rs. 2,16,000 Answer: Option C Explanation: Amount received from Company P after one year (i.e., in 199) on investing Rs. 12 lakhs in it = Rs. [12 + (8% of 12)] lakhs = Rs. 12.96 lakhs. Amount received from Company P after one year on investing Rs. 12.96 lakhs in the year 1999 = Rs. [12.96 + (10% of 12.96)] lakhs = Rs. 14.256. Appreciation received on investment during the period of two years = Rs. (14.256 - 12) lakhs = Rs. 2.256 lakhs = Rs. 2,25,600. 5.  An investor invested Rs. 5 lakhs in Company Q in 1996. After one year, the entire amount along with the interest was transferred as investment to Company P in 1997 for one year. What amount will be received from Company P, by the investor? A. Rs. 5,94,550 B. Rs. 5,80,425 C. Rs. 5,77,800 D. Rs. 5,77,500 Answer: Option B Explanation: Amount received from Company Q after one year on investment of Rs. 5 lakhs in the year 1996 = Rs. [5 + (6.5% of 5)] lakhs = Rs. 5.325 lakhs. Amount received from Company P after one year on investment of Rs. 5.325 lakhs in the year 1997 = Rs. [5.325 + (9% of 5.325)] lakhs = Rs. 5.80425 lakhs = Rs. 5,80,425. Study the following line graph which gives the number of students who joined and left the school in the beginning of year for six years, from 1996 to 2001. Initial Strength of school in 1995 = 3000. 1.  The number of students studying in the school during 1999 was? A. 2950 B. 3000 C. 3100 D. 3150 Answer: Option D Explanation: As calculated above, the number of students studying in the school during 1999 = 3150. 2.  For which year, the percentage rise/fall in the number of students who left the school compared to the previous year is maximum? A. 1997 B. 1998 C. 1999 D. 2000 Answer: Option A Explanation: The percentage rise/fall in the number of students who left the school (compared to the previous year) during various years are: For 1997 = (450 - 250) x 100 % = 80% (rise). 250 For 1998 = (450 - 400) x 100 % = 11.11% (fall). 450 For 1999 = (400 - 350) x 100 % = 12.5% (fall). 400 For 2000 = (450 - 350) x 100 % = 28.57% (rise). 350 For 2001 = (450 - 450) x 100 % = 0%. 450 Clearly, the maximum percentage rise/fall is for 1997. 3.  The strength of school incresed/decreased from 1997 to 1998 by approximately what percent? A. 1.2% B. 1.7% C. 2.1% D. 2.4% t 4.  The number of students studying in the school in 1998 was what percent of the number of students studying in the school in 2001? A. 92.13% B. 93.75% C. 96.88% D. 97.25% Answer: Option B Explanation: Required percentage = 3000 x 100 % = 93.75% 3200 5.  The ratio of the least number of students who joined the school to the maximum number of students who left the school in any of the years during the given period is? A. 7:9 B. 4:5 C. 3:4 D. 2:3 Answer: Option D Explanation: Required ratio = 300 = 2 . 450 3